
Mathuselahs and crosses.
 |
The Game
of Life: Mathuselahs and crosses. |
| Maths Workshop | |
| Mathuselahs. | |
|
Mathuselah patterns are those with less than 10 cells which do not become stable until after more than 50 generations. We have already focused on two of these patterns in earlier sections: the R-pentomino and Pi. The diagram below illustrates some of the most interesting Mathuselahs which have been discovered so far:
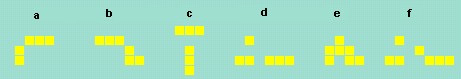
The first pattern, a, is the least known. It soon changes into the R-pentomino and becomes stable after 1,105 generations. The second pattern, b, stabilises after 608 generations. The thunderbird, c, lasts 243 generations and d 1,108 generations. The heptomino e becomes stable after 148 generations. However, the most surprising pattern is the last one, the acorn, which lives for 5,206 generations and stabilises as an oak tree consisting of 633 cells, after having produced numerous gliders. Watch its evolution in the following window: | |
| Crosses. | |
|
Another interesting group of patterns are the crosses. Investigate the life histories of 5-cell crosses, like the ones below:
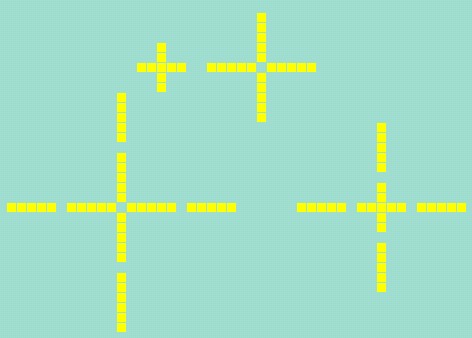
Use the window above to study their behaviour. |
| José Luis Alonso Borrego. | ||
 |
||
| Spanish Ministry of Education. Year 2001 | ||

Except where otherwise noted, this work is licensed under a Creative Common License